Movatterモバイル変換

[0]ホーム
URL:

Skip to main page content
U.S. flag

An official website of the United States government

Dot gov

The .gov means it’s official.
Federal government websites often end in .gov or .mil. Before sharing sensitive information, make sure you’re on a federal government site.

Https

The site is secure.
Thehttps:// ensures that you are connecting to the official website and that any information you provide is encrypted and transmitted securely.

NIH NLM Logo
Log inShow account info
Access keysNCBI HomepageMyNCBI HomepageMain ContentMain Navigation
pubmed logo
Advanced Clipboard
User Guide

Full text links

Atypon full text link Atypon Free PMC article
Full text links

Actions

Share

.2005 Mar 1;102(9):3318-23.
doi: 10.1073/pnas.0404782102. Epub 2005 Feb 22.

Mechanical feedback as a possible regulator of tissue growth

Affiliations

Mechanical feedback as a possible regulator of tissue growth

Boris I Shraiman. Proc Natl Acad Sci U S A..

Abstract

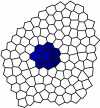
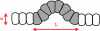
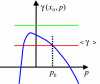
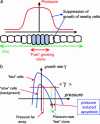
Regulation of cell growth and proliferation has a fundamental role in animal and plant development and in the progression of cancer. In the context of development, it is important to understand the mechanisms that coordinate growth and patterning of tissues. Imaginal discs, which are larval precursors of fly limbs and organs, have provided much of what we currently know about these processes. Here, we consider the mechanism that is responsible for the observed uniformity of growth in wing imaginal discs, which persists in the presence of gradients in growth inducing morphogens in spite of the stochastic nature of cell division. The phenomenon of "cell competition," which manifests in apoptosis of slower-growing cells in the vicinity of faster growing tissue, suggests that uniform growth is not a default state but a result of active regulation. How can a patch of tissue compare its growth rate with that of its surroundings? A possible way is furnished by mechanical interactions. To demonstrate this mechanism, we formulate a mathematical model of nonuniform growth in a layer of tissue and examine its mechanical implications. We show that a clone growing faster or slower than the surrounding tissue is subject to mechanical stress, and we propose that dependence of the rate of cell division on local stress could provide an "integral-feedback" mechanism stabilizing uniform growth. The proposed mechanism of growth control is not specific to imaginal disc growth and could be of general relevance. Several experimental tests of the proposed mechanism are suggested.

PubMed Disclaimer

Figures

Fig. 1.
Fig. 1.
A clone of cells carrying a certain somatic mutation forms a patch of a 2D tissue layer with different rate of cell growth and proliferation.
Fig. 2.
Fig. 2.
Schematic representation of a crosssection of a buckled region of a cell layer.
Fig. 3.
Fig. 3.
Pressure dependence of local tissue growth rate (the growth curve) and the feedback effect scenarios. Blue curve represents an assumed growth curve for a mutant clone. Negative γ represents apoptosis. In the case in which background growth rate (red line) is slower than the rate of clone growth, the growth-rate differential leads to the increase of local pressure within the clone until it reachesp0, at which the rates of growth for the clone and background equalize. For the case of growth-impaired clones, the rate of background growth (represented by the green line) can be higher than the maximal possible rate of clone growth. The growth-rate differential then leads to a decrease in local pressure and if blue and green curves do not intersect, the rate of growth in the clone cannot catch up with that of the background leading to continuing build-up of negative pressure (i.e., tension). Excessive tension in the tissue and the consequent distortion of cells may trigger apoptosis. This scenario could explain elimination of slow-growing clones by cell competition (6, 11).
Fig. 4.
Fig. 4.
Growth of clone size (defined as the number of cells in the clone) with time. (a) Expected time course of clone size. The average number of cells in a clone grows exponentially. Red indicates expected growth of a background clone. Blue indicates expected growth of a mutant clone with accelerated rate of proliferation in the presence of growth feedback. Initial rate of growth is faster than that of a background clone. At later stages, the rate of growth is reduced to match that of the background, resulting in a ratio of mutant to background clone sizes (the overgrowth ratio) independent of time. (b) Simulated time course of clone size for a stochastic cell division process modeled by two independent sequential random Poisson processes with equal rates. Blue lines represent different realizations of the random process. Note that most of the variance of the clone size distribution is acquired at early times when the clones have a small number of cells, but the variance of the size distribution does not decrease with time. Green and red lines represent realizations of the growth process with a linear integral feedback of increasing strength. Integral feedback causes the clones that have attained larger than average size early on to grow more slowly. This effect results in a decrease of variance of with time.
Fig. 5.
Fig. 5.
Nonautonomous effects of nonuniform growth and a possible scenario for cell-competition phenomenon. (a) Accelerated growth within an isolated clone causes compression of nearby tissue (and a distortion of cells decreasing in inverse proportion to the distance). (b) Growth curves for fast growing mutant clone cells (upper blue curve) and the slower-growing background tissue (lower blue curve). Red line represents the average growth rate of the background. Mutant clone overgrows until the resulting compression (black dashed line) brings its growth rate down to that of the background. Level of compression of WT tissue neighboring the clone (green dashed line) may be sufficiently high to trigger the apoptosis of the slower-growing WT cells near the clone.
See this image and copyright information in PMC

Similar articles

See all similar articles

Cited by

See all "Cited by" articles

References

    1. Sean, B. Carroll, S. W. & Grenier, J. (2000) From DNA to Diversity: Molecular Genetics and the Evolution of Animal Design (Blackwell Science, Oxford).
    1. Gerhart, J. & Kirschner, M. (1997) Cells, Embryos, and Evolution: Towards a Cellular and Developmental Understanding of Phenotypic Variation and Evolutionary Adaptability (Balckwell Science, Oxford).
    1. Day, S. J. & Lawrence, P. A. (2000) Development (Cambridge, U.K.) 127, 2977-2987. - PubMed
    1. Conlon, I. & Raff, M. (1999) Cell 96, 235-244. - PubMed
    1. Serrano, N. & O'Farrell, P. H. (1997) Curr. Biol. 7, R186-R195. - PMC - PubMed

Publication types

MeSH terms

Grants and funding

LinkOut - more resources

Full text links
Atypon full text link Atypon Free PMC article
Cite
Send To

NCBI Literature Resources

MeSHPMCBookshelfDisclaimer

The PubMed wordmark and PubMed logo are registered trademarks of the U.S. Department of Health and Human Services (HHS). Unauthorized use of these marks is strictly prohibited.

[8]ページ先頭
©2009-2025 Movatter.jp